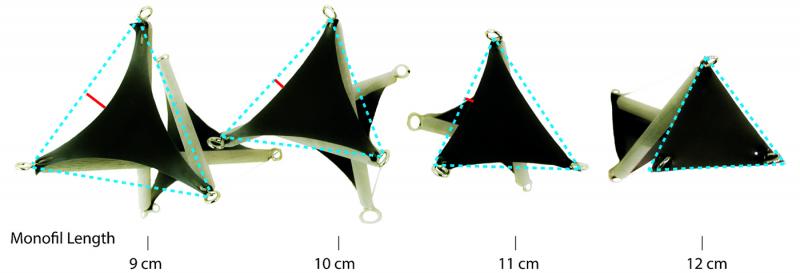
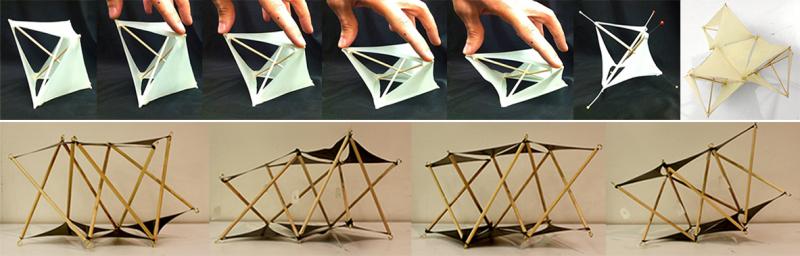
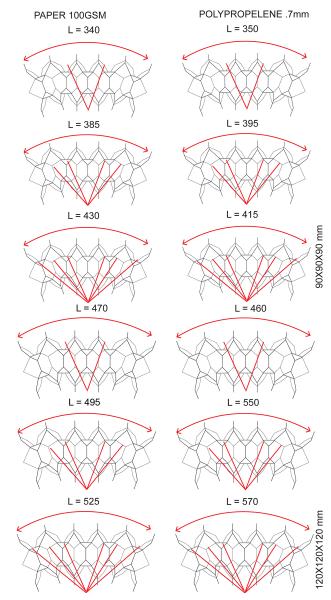
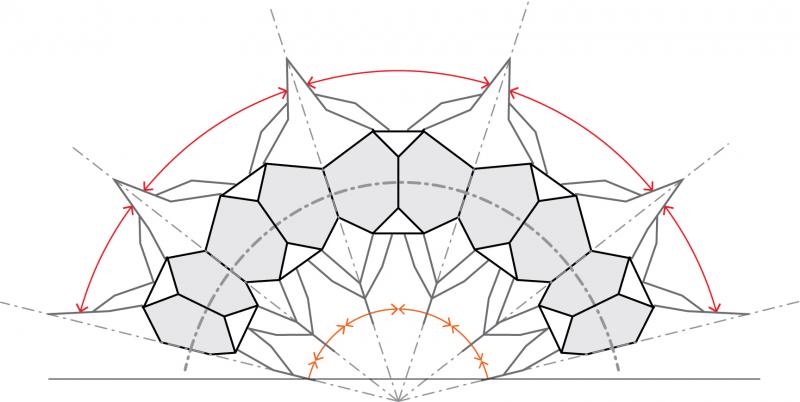
Crease
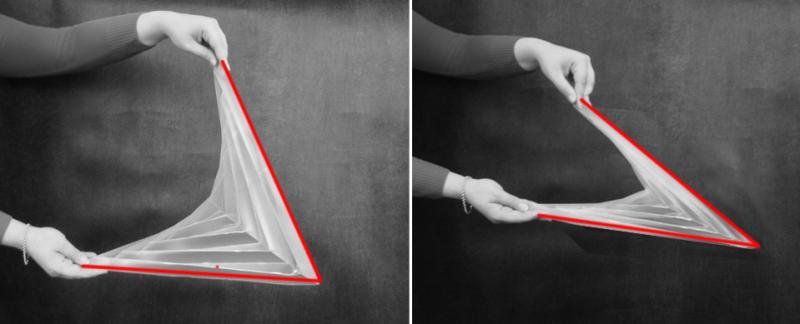
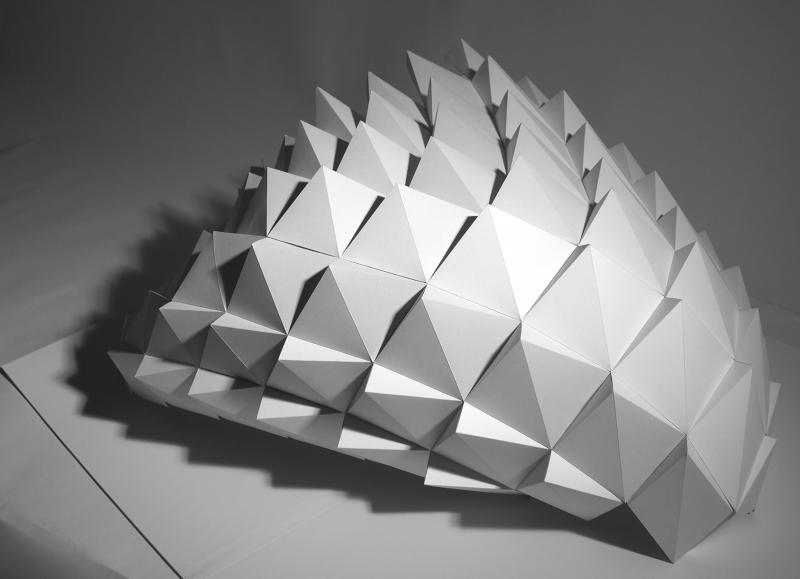
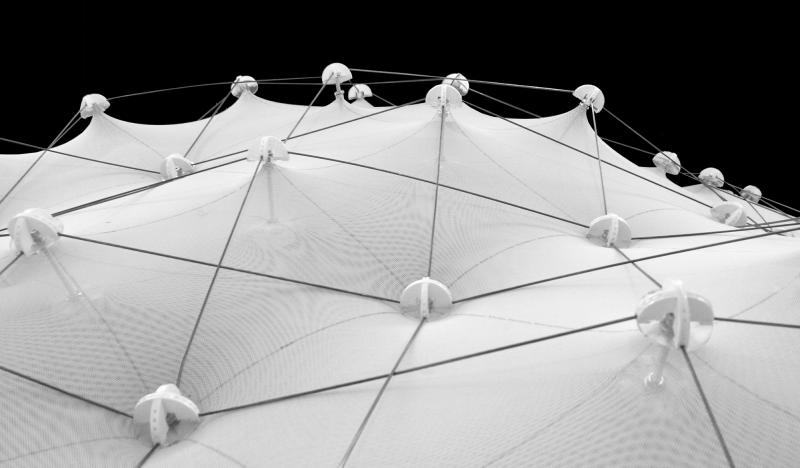
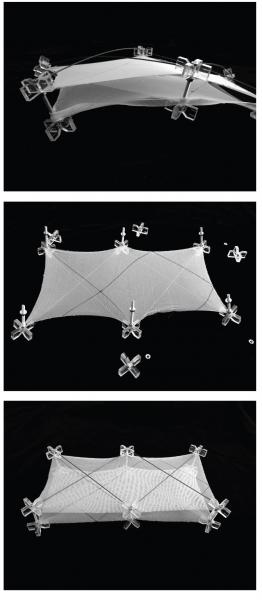
The base module for the investigation of a component-based material system was the hyperbolic parabola. The aim was to use this geometry to create a double curved surface. The main source of reference for the techniques of replicating this geometry were taken from origami folding methods, where paper was corrugated using mountain-valley folds which then created a double-curve unit. Due to the behaviour of paper, the geometry was able to shift, compress and expand.
Shahad Abdulmonem
Arghavan Arjmand Nik
Stanley Carroll
Alessandro Guidetti